I think the premise that inflation can be precisely measured is false. I know what you are probably thinking, you are probably thinking: "What do you mean you cannot precisely measure the inflation? Simply measure the average wages over time, plot them, and calculate the first derivative. The first derivative is the inflation.". Well, see, it isn't. To understand what I mean, consider this diagram of methane concentrations in the atmosphere over time:
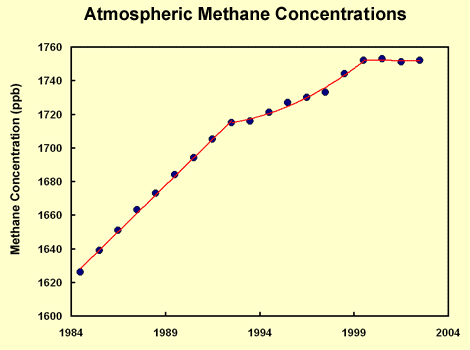
You are probably thinking "OK, our methane emissions have to be slightly higher than the derivative of that curve. It can be read from the diagram that our methane emissions reached their peak in the 1980s and have been decreasing ever since.". But that's wrong. I've made a video explaining why that way of thinking is wrong (I shall warn you that I assumed some substantial background knowledge of cybernetics):
https://youtu.be/EWI3wIJTZXg
However, like I mentioned in the video, that way of thinking seems to be common in social sciences. The Monetary School of Economics seems to take it for granted that one can easily determine what the inflation is and that the government can control it. For similar reasons, I now think the Skok's Third Law of toponymy is nonsense. My work in the science of the Croatian toponyms accidentally doesn't seem to suffer from that problem, but, like I've said, it seems to me that plenty of widely-accepted things in social sciences seem to do.
I was wondering what you think about that problem.